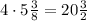Question

You are previewing this assessment x To multiply 4x5 the mixed number can be broken down into a whole number and a fraction in this case, that whole number would be choose y and the fraction would be Choose eighths Each of these parts are then Choose Choose Finally, these two are added together N

Answer 1

Given:

`4\cdot5(3)/(8)`

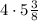
A mixed number is formed by a whole number and a fraction.

In this case, the given number is 5 3/8, the first part of the number is the whole number, and the second part is the fraction

So, for the given mixed number "5" is the whole number and "3/8" (three-eighths) is the fraction.

To solve the multiplication you can break down the number and multiply each part by 4:

`\begin{gathered} 4\cdot5(3)/(8) \\ \\ \end{gathered}`

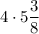
Multiply the whole number by 4:

`\to4\cdot5=20`

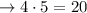
Multiply the fraction by 4:

`\to4\cdot(3)/(8)=(4)/(1)\cdot(3)/(8)=(4\cdot3)/(1\cdot8)=(12)/(8)`

Both "12" and "8" are multiples of 4, you can divide the numerator and denominator of the fraction by 4 to simplify the result:

`(12/4)/(8/4)=(3)/(2)`

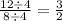
Once you solved both multiplications you have to add the products 20 and 3/2.

`20+(3)/(2)=20(3)/(2)`

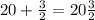
The result of the multiplication is then:

`4\cdot5(3)/(8)=20(3)/(2)`