Question

Find the length of the segment indicated. Round your answer to thenearest tenth if necessary.1 point18.2x17.1

Answer 1

We can note that the triangle on the right hand side is a right triangle. This implies that we can use Pytagorean theorem to find x, that is,

`x^2+17.1^2=18.2^2`

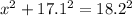
which gives

`x^2+292.41=331.24`

By moving 292.41 to the right hand side, we have

`\begin{gathered} x^2=331.24-292.41 \\ x^2=38.83 \end{gathered}`

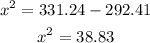
then, x is given by

`\begin{gathered} x=\sqrt[]{38.83} \\ x=6.23 \end{gathered}`

therefore, by rounding down, the answer is x=6.2 which corresponds to option 2.