Question

Trey is choosing between two exercise routines. In routine #1, he does only running, burning 8.5 calories per minute. In routine #2, he burns 17 calories walking. He then runs at a rate that burns 4.25 calories per minute. For what amounts of time spent running will routine #1 burn more calories then routine #2? Use t for the number of minutes spent running, and solve your inequality for t.

Answer 1

Let's use the variable y to represent the amount of calories burned.

For routine #1, we can write the equation:

`y_1=8.5\cdot t`

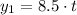
For routine #2, we have:

`y_2=17+4.25\cdot t`

If routine #1 will burn more calories, we have the inequality:

`\begin{gathered} y_1>y_2 \\ 8.5t>17+4.25t \\ 8.5t-4.25t>17 \\ 4.25t>17 \\ t>(17)/(4.25) \\ t>4 \end{gathered}`

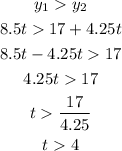
So for t > 4 (more than 4 minutes running) the routine #1 will burn more calories than routine #2.