Question

The two top concert tours in 2016 were concert A and concert B Based on iverage ticket prices, it cost a total of $1719 to purchase six tickets for concert A and ninetickets for concert B Four tickets for concert A and three tickets for concert B cost a total of 807 How much did an average ticket cost for each tour?

Answer 1

Explanation:

Given the information, create a system of equations.

Let x be the cost of each ticket for concert A

Let y be the cost of each ticket for concert B

If it cost $1,719 to purchase six tickets for concert A and nine tickets for concert B.

`6x+9y=1719\text{ \lparen1\rparen}`

If it cost $807 to purchase four tickets for A and three tickets for B.

`4x+3y=807\text{ \lparen2\rparen}`

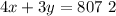
Isolate one of the variables, in this case ''x'' in one of the equations. Substitute x into the other equation.

Isolate x in (2).

`\begin{gathered} x=(807)/(4)-(3)/(4)y \\ x=-(3)/(4)y+201.75 \end{gathered}`

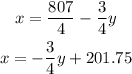
Plug it into equation (1):

`\begin{gathered} 6(-(3)/(4)y+201.75)+9y=1719 \\ -(9)/(2)y+1210.5+9y=1719 \\ 4.5y=1719-1210.5 \\ y=(508.5)/(4.5) \\ y=\text{ \$113} \end{gathered}`

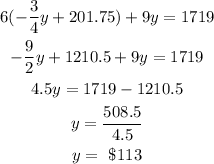
Knowing the value of ''y'', substitute it into the equation (2):