Question

Determine the slope of any line perpendicular to the line illustrated in the graph

Answer 1

We are asked to find the slope of a line perpendicular to the line shown in the graph.

To find this desired slope, we must remember that if you multiply the slopes of two perpendicular lines in the plane, you get -1. That is, the slopes of the perpendicular lines are opposite reciprocals.

That is, multiplying the slope of two perpendicular lines should result in -1.

Now let's find the slope of the line on the graph with the following equation:

`m=\frac{y_2-y_{1_{}}}{x_2-x_1}`

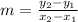
Where

`\begin{gathered} (x_1,y_1)=(1,-4) \\ (x_2,y_2)=(2,-1) \end{gathered}`

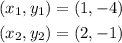
Now, we replace and solve the slope

`\begin{gathered} m=(-1-(-4))/(2-1) \\ m=(-1+4)/(2-1) \\ m=(3)/(1) \\ m=3 \end{gathered}`

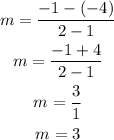
In conclusion, the slope of the line in the graph is m=3

Now we solve the slope of the perpendicular line:

`\begin{gathered} m\cdot m_p=-1 \\ 3\cdot-(1)/(3)=-1 \end{gathered}`

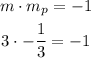
In conclusion, the answer is -1/3