Given the inequality, we first can look at the intercepts in the x-axis and the y-axis. We can find them like this:
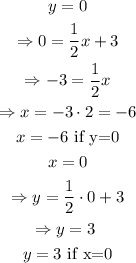
Now that we have the two points (-6,0) and (0,3), we can make the graph of the line:
Now, to determine the inequality, we look at the point (0,0) and use it on the inequality:
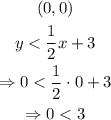
Since we found that the point (0,0) fits in the inequality, this must mean that the point (0,0) must be on the side of the solution, therefore, the graph of the inequality would be like this: