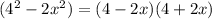Question

I got to the final step where I need to simplify the expression but I don’t know how to

Answer 1

The simplify an expression as a frection, we need to find eqaul terms in the nominator and denominator, to cancell them. The idea is, if we have a number called n, and a expression like:

`(nx)/(ny)`

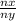
We can divide n by n in order to get a simplifyed expression.

In this case we have:

`(d)/(dx)((-4x^2+16)/((x^2+4)^2))=((x^2+4)^2\cdot(-8x)-4x(x^2+4)(-4x+16))/((x^2+4)^4)`

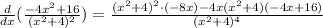
We can see that the term (x² + 4) is repeated in the numerator and denominator. To do this easier, let's separate this in a sum of fractions:

`((x^2+4)^2\cdot(-8x)-4x(x^2+4)(-4x+16))/((x^2+4)^4)=((x^2+4)^2\cdot(-8x))/((x^2+4)^4)-(4x(x^2+4)(-4x+16))/((x^2+4)^4)`

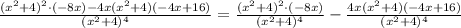
Now it's much easier to cancell the repeated terms:

In the first fraction, we have the parentheses squared in the numerator and power of 4 in the denominator, to divide it, we can use the power propierties:

`(a^n)/(a^m)=a^(n-m)`

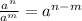
Then:

`((x^2+4)^2)/((x^2+4)^4)=(x^2+4)^(2-4)=(x^2+4)^(-2)=(1)/((x^2+4)^2)`

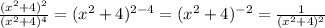
And now the first fraction is:

`((x^2+4)^2\cdot(-8x))/((x^2+4)^4)=(1)/((x^2+4)^2)\cdot(-8x)=-(8x)/((x^2+4)^2)`

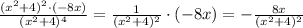
For the second fraction is very similar:

`(4x(x^2+4)(-4x+16))/((x^2+4)^4)`

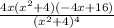
We have the same term (x² + 4) , in the numerator and denominator. Then divide:

`((x^2+4))/((x^2+4)^4)=(x^2+4)^(1-4)=(x^2+4)^(-3)=(1)/((x^2+4)^3)`

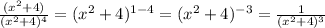
Then the second fraction is:

`4x(-4x+16)\cdot(1)/((x^2+4)^3)=(4x(-4x^2+16))/((x^2+4)^3)`

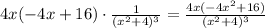
Now we can add the two fraction to get the final asnwer:

`(d)/(dx)((-4x^2+16)/((x^2+4)^2))=-(8x)/((x^2+4)^2)-(4x(-4x^2+16))/((x^2+4)^3)`

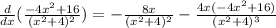
And that's all the simplifying we can do with this derivative

If we look at the second fraction, we have a difference of squares:

`(16-4x^2)=(4^2-2x^2)`

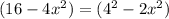
Thus:

`(4^2-2x^2)=(4-2x)(4+2x)`