We can find the equation of a line given one point and its slope.
Remember that two parallel lines have the same slope; therefore, the slope of 5x-2y-4=0 is equal to the slope of the line we are trying to find.
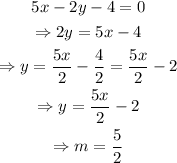
Then, we have got everything we need, the slope is equal to 5/2 and a point in the line is (2,-4)
The equation is:
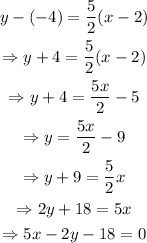
The answer is 5x-2y-18=0