This question is asking about the value of the angle that gives us a sine of 4/15.
To find this angle, we need to use the inverse function of the sine function which is called arcsin function, and it is also represented as:
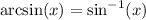
Then, to find the angle, we need to apply the latter function on both sides of the equation as follows:
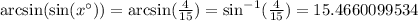
We need to be careful that the value that gives us a calculator is in degrees (as in this case).
If we round the answer to the nearest tenth, we finally have that:
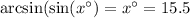
In summary, the value for angle x° is equal to 15.5° (third option).