Question

4. The distribution of the heights of students in a large class is roughly Normal. The average height is 68inches, and approximately 99.7% of the heights are between 62 and 74 inches. Thus, the variance of theheight distribution is approximately equal to(A) 2(B) 3(C) 4D 6(E) 9

Answer 1

Step 1: Let's recall the 68 - 95 - 99.7 rule for a standard normal distribution, as follows:

• 68% of data falls within the first standard deviation from the mean

,

• 95% of data falls within the second standard deviation from the mean

,

• And, finally 99.7% of data falls within the third standard deviation from the mean

Step 2: Now, let's calculate the value of the standard deviation for the heights of students in this large class, this way:

3 * Standard deviation = Upper limit - Mean

Replacing with the values given in the exercise, we have:

3 * Standard deviation = 74 - 68

3 * Standard deviation = 6

Dividing by 3 at both sides:

3 * Standard deviation/3 = 6/3

Standard deviation = 2 inches

Step 3: Let's also recall that the standard deviation of a sample or of a population is equal to the square root of the variance. Then, using that formula, let's find the variance for this normal distribution.

Standard deviation = √Variance

2 = √Variance

Squaring to both sides:

`2^2\text{ = (}√(Variance))^2`

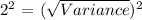
Variance = 4 square inches

The correct answer is C. 4