Question

Am I correct? I just want to know if I got it correct.

Answer 1

We want to solve the following equation

`x^2-12x+36=80`

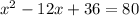
we begin by subtracting 80 on both sides, so we get

`x^2-12x+36-80=x^2\text{ -12x -44}=0`

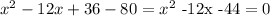
So we have the equivalent problem of solving the equation

`x^2\text{ -12x-44=0}`

Recall that having an equation of the form

`ax^2+bx+c=0^{}`

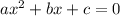
the solutions are given by the equation

`x=\frac{\text{ -b }\pm\sqrt[]{b^2\text{ -4ac}}}{2a}`

In our case we have a=1, b=-12 and c= -44 so the solutions are

`x=\frac{\text{ -(-12)}\pm\sqrt[]{(-12)^2\text{ -4(1)( -44)}}}{2(1)}=\frac{12\pm\sqrt[]{320}}{2}=\frac{12\pm8\sqrt[]{5}}{2}=6\pm4\sqrt[\square]{5}`

so the solutions to the original problem are

`x=6+4\sqrt[]{5}`

and

`x=6\text{ -4}\sqrt[]{5}`