To answer this question, we can proceed as follows:
1. We have that the perimeter of the rectangle is equal to 116cm, then, we have:

We know that a rectangle is a parallelogram. Then, its opposite sides are congruent.
2. We have that the length of the rectangle is 1 cm more than twice its width. We can translate this, algebraically, as follows:
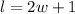
Now, to find the measures of the length and the width of the rectangle, we can substitute this last formula into the first one, as follows:
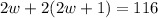
We need to apply the distributive property to find w:
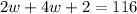
Adding like terms:
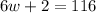
Subtracting 2 to both sides of the equation, and then dividing by 6:
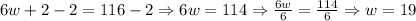
Then, the width of the rectangle is equal to 19cm. The measure of the length can be calculated using either equation above. Let us use the first equation:
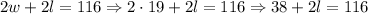
Then, using similar properties as before, we have:
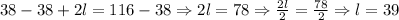
In summary, we have that the measures of the length and width of this rectangle are:
• Width, ,(w) =, 19cm
,
• Legth (l) = ,39cm