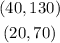We are given the following system of equations:
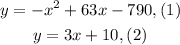
We will substitute the value of "y" from the first equation into the second equation:

Now we subtract "3x" from both sides:
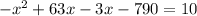
Adding like terms:
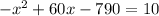
Now we subtract 10 from both sides:
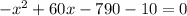
Adding like terms:
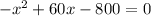
Now we multiply by -1 on both sides of the equation:
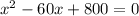
Now we factor in the left side. We need two numbers that when multiplied the product is 800 and their algebraic sum is -60. Those numbers are -40 and -20. Therefore, we get:
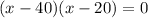
Now we set each factor to zero:

For the next factor:

These are the two values of "x" for the solution. To get the corresponding value of "y" we substitute in the second equation. Substituting the first value we get:
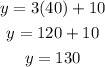
Now we substitute the second value:
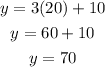
Therefore, the solutions of the system are: