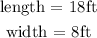Question

The length of a rectangle is 2ft more than twice the width. The area is 144 ft squared. Find the length and width of the rectangle.

Answer 1

The formula for the area of a rectangle (A) is given as

`A=\text{length}* breadth`

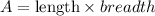
Given that

The length of a rectangle is 2ft more than twice the width,

Therefore,

length = 2 + 2width

where,

`\begin{gathered} w=\text{width} \\ l=2+2w \\ A=144ft^2 \end{gathered}`

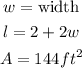
Hence,

`\begin{gathered} 144=(2+2w)* w \\ 144=2w+2w^2 \\ 144=2(w+w^2) \\ (144)/(2)=(2(w+w^2))/(2) \\ 72=w+w^2 \\ w^2+w-72=0 \end{gathered}`

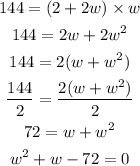
Factorizing the equation above

`\begin{gathered} w^2+9w-8w-72=0 \\ w(w+9)-8(w+9)=0 \\ (w-8)(w+9)=0 \\ w-8=0\text{ or }w+9=0 \\ w=8\text{ or w=-9} \\ \therefore w=8or-9 \end{gathered}`

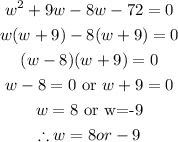
Note that the width can never be negative, therefore the width of the rectangle is 8.

Recall that:

`\begin{gathered} l=2_{}+2w=2+2(8)=2+16=18 \\ l=18 \end{gathered}`

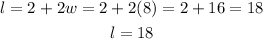
Hence,

`\begin{gathered} \text{length = 18ft} \\ \text{width = 8ft} \end{gathered}`