Given:
The mass of block 1, m₁=2 kg
The mass of the block 2, m₂=5 kg
The initial velocity of the block 1, u₁=3 m/s
The initial velocity of the block 2, u₂=0 m/s
The velocity of the block 2 after the collision, v₂=1.5 m/s
To find:
The magnitude and direction of the velocity of block 2 after the collision.
Step-by-step explanation:
From the law of conservation of momentum, the total momentum of blocks before the collision must be equal to the total momentum of the blocks after the collision.
Thus,
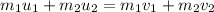
Where v₁ is the velocity of block 1 after the collision.
On rearranging the above equation,
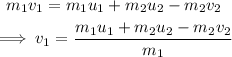
On substituting the known values,
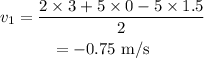
The negative sign indicates that block 1 will be sliding to the left after the collision.
Final answer:
Thus the magnitude of the velocity of block 1 after the collision is 0.75 m/s.
And the direction of block 1 after the collision is to the left.