ANSWER
The solution is (8, -2)
Step-by-step explanation
The substitution method consists in solving one of the equation for one of the variables - it will be as a function of the other variable, then substitute that variable by this expression into the other equation. There we'll have an equation for one of the variables, we solve that and the substitute the value into the expression we found first.
For this problem let's solve the first equation for y:

We can just add y to both sides of the equation and then subtract 58 from both sides:
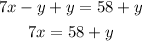
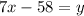
Now we substitute y by this expression into the second equation:

And solve for x. First apply the distributive property to the second term:

Add like terms - this means adding the coefficients of x:
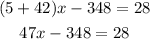
Then add 348 to both sides of the equation:
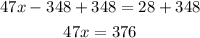
Finally, divide both sides by 47:
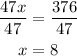
Now, to find y we just have to substitute x = 8 into the expression we found for y as a function of x:
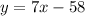

So the solution to the equation is x = 8 and y = -2, which is the point (8, -2)