Complex numbers can be written in two forms:
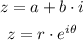
Where a and b are known as the real and the imaginary part and r and theta are the magnitude and the angle of the number. In this case we are given these last two quantities and we have to find a and b. One way to do this is recalling an important property of the exponential expression above:
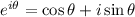
Then the exponential form of a number is equal to:
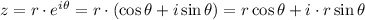
And since we are talking about the same number then this expression must be equal to that given by a and b:
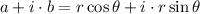
Equalizing terms without i and those with i we have two equations:
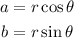
Now let's use the data from the exercise:
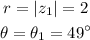
Then we have:
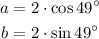
Using a calculator we can find a and b:
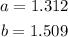
Then the answers for the two boxes are 1.312 and 1.509