Given:
Measure of each interior angle = 120 degrees
Length of each side = 4.6 cm
Let's find the perimeter of the polygon.
Since the measure of each interior angle is 120 degrees, let's find the number of sides of the polygon using the formula below:
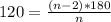
Let's solve for n.
We have:

Divide both sides by 60:
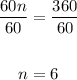
Therefore, the polygon has 6 sides.
To find the perimeter, apply the formula:
Perimeter = number of sides x length of each side
Perimeter = 6 x 4.6
Perimeter = 27.6 cm
Therefore, the perimeter of the polygon is 27.6 cm
ANSWER:
27.6 cm