Given,
The initial velocity of the car, u=13.6 m/s
The final velocity of the car, v=0 m/s
The distance covered by the car, d=0.321 m
From the equation of the motion,
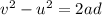
On rearranging the equation,
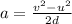
This is the acceleration of the car which brings the car to rest after the collision.
On substituting the known values,
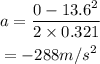
From another equation of the motion,
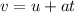
On rearranging the above equation,
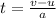
On substituting the known values,
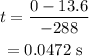
Thus the car comes to stop in 0.0472 seconds.