Question

a tank in the shape of a sphere is filled with water and has a diameter of 15ft. if water weighs 62.4 pounds per cubic foot what is the total weight of the water in a full tank to the nearest pound

Answer 1

111,270 pounds ndjskssksjsbahahahaahahababahahahahahahaha

Answer 2

110,270 pounds.

Step-by-step explanation:

Diameter of the Spherical Tank = 15 ft

• Radius = 15 ÷ 2 = 7.5 ft

For any sphere with radius, r:

`\text{Volume}=(4)/(3)\pi r^3`

Therefore, the volume of water that will fill the tank:

`\begin{gathered} V=(4)/(3)*\pi*7.5^3 \\ =1767.15\; ft^3 \end{gathered}`

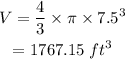
If water weighs 62.4 pounds per cubic foot:

`\begin{gathered} \text{Density}=\frac{\text{Weight}}{\text{Volume}} \\ 62.4=\frac{\text{Weight}}{1767.15} \\ \text{Weight}=62.4*1767.15 \\ \text{Weight}=110,270.16\text{ pounds} \\ \text{Weight=}110,270\text{ pounds} \end{gathered}`

The total weight of the water in a full tank is 110,270 pounds. (correct to the nearest pound).