Hello, I am currently very stuck with this problem and I am unsure as to how I would solve it.

Question

asked Sep 17, 2023 39.5k views

1 Answer

Todd Sjolander · Answer 1 · 2023-09-23T15:34:08+0000

We have the equation

Let's complete the square, to do it let's add and subtract 25 on the right side

$\begin{gathered} 20y=x^2-10-15+25-25 \\ \\ 20y=(x-5)^2-15-25_{} \\ \\ 20y=(x-5)^2-40 \\ \\ \end{gathered}$

Now we can have y in function of x

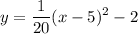
$\begin{gathered} y=(1)/(20)(x-5)^2-2 \\ \\ \end{gathered}$

Now we can already identify the vertex because it's in the vertex form:

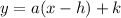
Where the vertex is

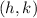
As we can see, h = 5 and k = -2, then the vertex is

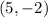
Now we can continue and find the focus, the focus is

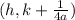
$\mleft(h,k+(1)/(4a)\mright)$

We have a = 1/20, therefore

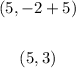
$\begin{gathered} \mleft(5,-2+5\mright) \\ \\ (5,3) \end{gathered}$

The focus is

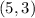
And the last one, the directrix, it's

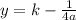
Then

$\begin{gathered} y=-2-5 \\ \\ y=-7 \end{gathered}$

Hence the correct answer is: vertex (5, -2); focus (5, 3); directrix y = -7