Solution:
Given:
Let the amount of Type 1 nitric acid used be represented by a
Let the amount of Type 2 nitric acid used be represented by b
Hence, the system of equations is;

Thus, solving the system of equations simultaneously,
From equation (1),

Solving for a,
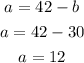
Therefore, the answer is;
12 grams of Type 1 and 30 grams of Type 2