Question

hi! I have the answers to A. AND B. which are k=1.243% for a and k= 0.20% for bWhere y is the population after t time, A is the initial population and k is the growth constant.Therefore, for each case, we calculate the value of k:(a)t = 4y = 1375000A = 1309000Solving for k:1375000=1309000⋅ek⋅4e4k=137500013090004k=ln(13750001309000)k=ln(13750001309000)4k=0.01229≅0.0123→1.23%(b)t = 4y = 1386000A = 1375000Solving for k:1386000=1375000⋅ek⋅4e4k=138600013750004k=ln(13860001375000)k=ln(13860001375000)4k=0.00199≅0.002→0.20%(c)To compare we calculate the quotient between both periods:

Answer 1

The population growth rate formula is given as

`P=P_0e^(rt)`

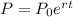
Where P is the final population

Po= is the initial population

P is the final population

r is the rate

t is the time taken

If it has been calculated that the growth rate from 2012 to 2016 is 1.23% and from 2016 to 2020 is 0.20%

(c) From these two growth rates, it can be seen that 1.23% is greater than 0.20%, we can conclude that the growth rate from 2012 to 2016 is greater than the growth rate from 2016 to 2020

(d) If the current growth rate continues,, the time it will take for the population to reach 1.5million is as shown below:

`\begin{gathered} P=1500000 \\ P_0=1386000 \\ r=0.20 \\ t=\text{?} \\ P=P_0e^(rt) \\ P=P_0e^(0.2t) \end{gathered}`

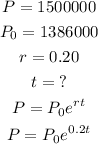
This becomes

`\begin{gathered} 1500000=1386000e^(0.2* t) \\ (1500000)/(1386000)=e^(0.2t) \\ 1.08225=e^(0.2t) \\ \ln e^(0.2t)=\ln 1.08225 \\ 0.2t=\ln 1.08225 \end{gathered}`
`\begin{gathered} t=(\ln 1.08225)/(0.2) \\ t=(0.0790432)/(0.2)=0.395 \end{gathered}`

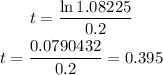
Answer Summary

(a) The growth rate from 2012 to 2016 is 1.23%

(b) The growth rate from 2016 to 2020 is 0.20%

(c) In comparison, the growth rate from 2012 to 2016 is greater than the growth rate from 2016 to 2020

(d) The time it will reach the 1.5 million if the current growth rate continues is 0.395years