Question

SA bag contains 1 gold marbles, 6 silver marbles, and 21 black marbles. Someone offers to play this game: Yourandomly select one marble from the bag. If it is gold, you win $3. If it is silver, you win$2. If it is black, youlose $1.What is your expected value if you play this game?

Answer 1

We are given that a bag contains 1 gold marble, 6 silver marbles, and 21 black marbles. First, we need to determine the total number of marbles. The number of marbles of each color is:

`\begin{gathered} N_(gold)=1 \\ N_(silver)=6 \\ N_{\text{black}}=21 \end{gathered}`

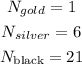
The total number is then:

`N_t=N_{\text{gold}}+N_{\text{silver}}+N_{\text{black}}`

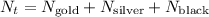
Substituting the values:

`N_t=1+6+21=28`

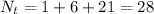
Therefore, there are a total of 28 marbles. Now we determine the probability of getting each of the marbles by determining the quotient of the number of marbles of a given color over the total number of marbles. For the gold marbles we have:

`P_{\text{gold}}=\frac{N_{\text{gold}}}{N_t}=(1)/(28)`

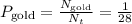
For silver we have:

`P_{\text{silver}}=(N_(silver))/(N_t)=(6)/(28)=(3)/(14)`

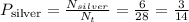
For the black marbles:

`P_{\text{black}}=\frac{N_{\text{black}}}{N_t}=(21)/(28)=(3)/(4)`

Now, to determine the expected value we need to multiply each probability by the value that is gained for each of the colors. We need to have into account that is it is a gain we use a positive sign and if it is a lose we use a negative sign:

`E_v=(3)((1)/(28))+(2)((3)/(14))+(-1)((3)/(4))_{}`

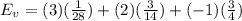
Solving the operations we get:

`E_v=-0.21`

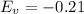
Therefore, the expected value is -$0.21.