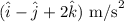Given,
The initial position of the boat, d_i=(5i-2j+4k) m
The initial velocity of the boat, u=(4i+j-3k) m/s
The time period, t=8 s
The final velocity of the boat, v=(12i-7j+13k) m/s
To find:
a) The position of the object after 8 s
b) The acceleration of the object.
Step-by-step explanation:
a)
From the equation of motion, the total distance traveled by the object is given by,
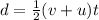
On substituting the known values,
![\begin{gathered} d=(1)/(2)[(12\hat{i}-7\hat{j}+13\hat{k)}+(4\hat{i}+\hat{j}-3\hat{k})]8 \\ =4*(16\hat{i}-6\hat{j}+10\hat{k}) \\ =(64\hat{i}-24\hat{j}+40\hat{k})\text{ m} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/3hs1oiu6ww0gyyss94kcrcbws33eiee0ya.png)
Thus the final position of the boat is,
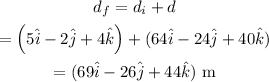
b)
The acceleration of an object is given by,
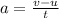
On substituting the known values,
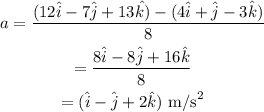
Final answer:
a) Thus the position of the boat after 8 s is
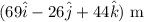
The acceleration of the boat is