Question

Frank has a circular Garden the area of the garden is 100 ft Square what is the approximate distance from the edge of Frank's garden to the center of the garden (A= 3.14r ² )

Answer 1

`5.64ft`

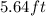
Step-by-step explanation

The approximate distance from the edge of the garden to the center is the radius of the garden.

The area of a circle is given as:

`A=\pi\cdot r^2`

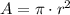
We can find the radius by making r the subject of the formula:

`\begin{gathered} (A)/(\pi)=(\pi\cdot r^2)/(\pi) \\ r^2=(A)/(\pi) \\ r=\sqrt[]{(A)/(\pi)} \end{gathered}`

Therefore, the approximate distance from the edge of the garden to the center (radius) is:

`\begin{gathered} r=\sqrt[]{(100)/(3.14)} \\ r=\sqrt[]{31.85} \\ r\approx5.64ft \end{gathered}`

That is the answer.