Question

I need help with 5 and 6. The exponent for part 5 if you can't see it well 2/3

Answer 1

5.

Given the equation to solve for x:

`3(x+1)^{(2)/(3)}=12`

The steps for the solution are as follows:

`\begin{gathered} 3(x+1)^{(2)/(3)}=12 \\ \frac{3(x+1)^{(2)/(3)}}{3}=(12)/(3) \\ (x+1)^{(2)/(3)}=4 \\ \lbrack(x+1)^{(2)/(3)}\rbrack^{(1)/(2)}=(4)^{(1)/(2)} \\ \lbrack(x+1)^{(1)/(3)}\rbrack=\pm2 \\ \lbrack(x+1)^{(1)/(3)}\rbrack^3=(\pm2)^3 \\ x+1=\pm8 \end{gathered}`

From the above equation, we have x + 1 = 8 and x + 1 = -8. These imply x = 7 and x = -9.

Check for extraneous solutions:

If x = 7, then the left-hand side of the equation is:

`3(x+1)^{(2)/(3)}=3(7+1)^{(2)/(3)}=3(4)=12`

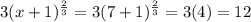
Thus, the equation holds true at x = 7.

If x = -9, then the right-hand side of the equation is:

`3(x+1)^{(2)/(3)}=3(-9+1)^{(2)/(3)}=3(4)=12`

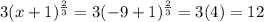
Thus, the equation holds true at x = -9.

There is no extraneous solution. The solutions of the given equation are x = 7 and x = -9.

6.

Given an equation to solve for x:

`\sqrt[]{3x+2}-2\sqrt[]{x}=0`

The steps of the solution are as follows:

`\begin{gathered} \sqrt[]{3x+2}-2\sqrt[]{x}=0 \\ \sqrt[]{3x+2}=2\sqrt[]{x} \\ (\sqrt[]{3x+2})^2=(2\sqrt[]{x})^2 \\ 3x+2=4x \\ 2=4x-3x \\ 2=x \end{gathered}`

Thus, the solution of the equation is x = 2.