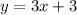Question

Find the equation of the linear function represented by the table below in slope-intercept form.X1234y691215******

Answer 1

Given:

Given a table.

Required:

To find the equation of the linear function.

Step-by-step explanation:

From the table

`\begin{gathered} (x1,y1)=(1,6) \\ (x2,y2)=(2,9) \end{gathered}`

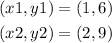
The general form of equation is

`y=mx+b`

Here the slope is

`\begin{gathered} m=(y2-y1)/(x2-x2) \\ \\ =(9-6)/(2-1) \\ \\ =(3)/(1) \\ \\ =3 \end{gathered}`

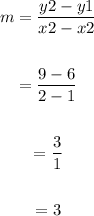
So

`y=3x+b`

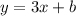
Now we have to find the value of b, by using the point (1,6)

`\begin{gathered} 6=3(1)+b \\ \\ 6-3=b \\ \\ b=3 \end{gathered}`

Now

`y=3x+3`

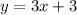
Final Answer:

The linear equation is

`y=3x+3`