ANSWER:
D.
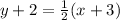
Step-by-step explanation:
Given:
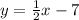
Recall that the slope-intercept form of the equation of a line is generally given as;

where;
m = slope of the line
b = y-intercept of the line
Comparing both equations above, we can see that the slope(m) of the line is 1/2 and the y-intercept(b) is -7
Recall that parallel lines have the same slope. So the line that is parallel to the given line will have the same slope(m) of 1/2
Given the point (-3, -2), we can go ahead and write the equation of the parallel line in point-slope form as seen below;
![\begin{gathered} y-y_1=m(x-x_1) \\ y-(-2)=(1)/(2)[(x-(-3)] \\ y+2=(1)/(2)(x+3) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/9qmb0x1ni66twj66qfj38hv9zp6qjd1o3k.png)