Question

Find an equation of the line passing through point (4, -5) and perpendicular to the line whose equation is y= 1/2x + 11/6. *In Slope-Intercept Form

Answer 1

Step-by-step explanation:

Given the equation:

`y=(1)/(2)x+(11)/(6)`

Comparing it with the slope-intercept form: y=mx+b

`\text{Slope,m}=(1)/(2)`

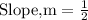
Definition: Two lines are perpendicular if the product of the slopes is -1.

Let the slope of the new line = n

`\begin{gathered} (1)/(2)n=-1 \\ n=-2 \end{gathered}`

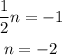
Substitute the slope, -2 and point (4,-5) in the slope-point form:

`\begin{gathered} y-y_1=m(x-x_1) \\ y-(-5)=-2(x-4) \end{gathered}`

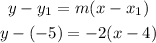
We then express it in the slope-intercept form:

`\begin{gathered} y+5=-2x+8 \\ y=-2x+8-5 \\ y=-2x+3 \end{gathered}`

The equation of the perpendicular line is y=-2x+3.

Answer:

`y=-2x+3`