Question

I need help with this trigonometric function I will upload a photo

Answer 1

For us to be able to determine the distance along an arc on the surface of the earth, we will be using the following formula:

`\text{ S = r}\theta`

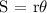
Where,

S = arc length

r = radius (radius of the earth)

θ = central angle (in radian)

Given:

r = 3960 miles

θ = 48 mins.

a.) Let's convert the given measure of the central angle to radian.

`\theta=48mins.\text{ = (48 mins.) x }\frac{1^(\circ)}{(60\text{ mins.})}\text{ = }(48)/(60)(1^(\circ))`
`\theta\text{ = }(4)/(5)^(\circ)`
`\text{ }\theta_(radian)\text{ = }\theta_(degrees)\text{ x }(\pi)/(180^(\circ))`
`\text{ }\theta_(radian)\text{ = }(4)/(5)\text{ x }(\pi)/(180)\text{ = }(4\pi)/(900)\text{ = }(\pi)/(225)\text{ radians}`

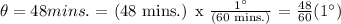
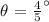
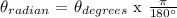
b.) Let's now determine the distance (arc length).

`\text{ S = r}\theta`
`\text{ S = (3960)(}(\pi)/(225)\text{ ) = }(3960\pi)/(225)\text{ miles = 17.6}\pi\text{ miles = 55.2920307 }\approx\text{ 55.292 miles}`

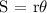
Therefore, the answer is 55.292 miles.