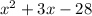
Let's solve for x by factoring this quadratic equation.
We first need to determine which two numbers multiply to -28 (c) but add to 3 (b).
The two numbers that multiply to -28 and add to 3 are 7 and -4.
Therefore, we can factor this equation like so:

Now, we can set this equation to 0 and solve for x.
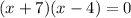
If we look at each factor individually, we can determine the values of x:

Subtract 7 from both sides of the equation.
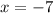
Now, let's look at the other factor:
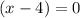
Add 3 to both sides of the equation.
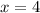
The solution of x^2 + 3x - 28 is x = -7, x = 4.