Question

What would be the correct way to solve this?
`{x}^(2) - 5x - 84`

Answer 1

(x + 7 ) ( x - 12 )

Explanation:

We know that if we multiply any two expressions x + a and x + b then we have

`(x+a)(x+b)=x^2+(a+b)x+ab`

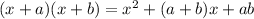
Now similarly,

`x^2+(a+b)x+ab=x^2-5x-84`

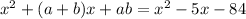
meaning

`\begin{gathered} a+b=-5 \\ ab=-84 \end{gathered}`

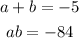
In other words, what are the two numbers that if I add them together I get -5 and If I multiply them I get -84. The answer comes from educated guesses. We guess that if we add 7 and -12 we get 5 and if we multiply then we get -84; therefore,

`\begin{gathered} a=7 \\ b=-12 \end{gathered}`

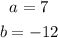
Hence, the expression can be factored as

`=x^2-5x-84=(x-12)(x+7)`

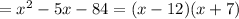
which is our answer!