Question

GIVEN: P(N) = 0.25 and P(R) = 0.6If the probability of P(N R) = 0.15, are N and Rindependent events?a) Yes, because P(N) + P(R) +0.15b) No, because P(N).P(R) +0.15c) Yes, because P(N) X P(R) = 0.15d) Not enough information

Answer 1

P(N∩R) represents the probability of A and B.

When two events are independent events, the joint probability is calculated by multiplying their individual probabilities.

P(N∩R) For independent events:

`P\mleft(N\cap R\mright)=P(N)* P(R)`

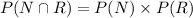
Substituting the known values for P(N) and P(R):

`\begin{gathered} P(N\cap R)=0.25*0.6 \\ P(N\cap R)=0.15 \end{gathered}`

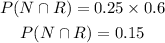
0.15 is the value of P(N∩R) given by the problem, and since we get the same result using the formula for independent events, we can affirm that N and R independent events.

Answer:

c) Yes, because P(N) X P(R) = 0.15