Question

You invest $275 to start a sandwich stand and decide to charge $5.15 per sandwich.Set up a Linear Model that determines your profit or loss based on the number of sandwiches.How much money will you make if you sell 75 sandwiches?How many sandwiches must you sell to make a $100 profit?

Answer 1

Explanations:

Let the number of sandwichs sold be "x"

If you charge $5.15 per sandwich then the total sales of the sandwich will be 5.15x

Cost price = $275

The Linear Model that determines your profit or loss based on the number of sandwiches will be expressed as:

`\text{Profit}=\text{Selling price - Cost price}`

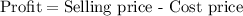
Substitute the given parameters;

`\begin{gathered} \text{Profit/Loss}=5.15x\pm275 \\ p(x)=5.15x\pm275 \end{gathered}`

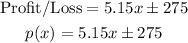
If 75 sandwiches were sold, the amount of money made will be expressed as:

`\begin{gathered} p(75)=5.15(75)-275 \\ p(75)=386.25-275 \\ p(75)=\$111.25 \end{gathered}`

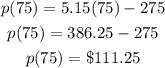
Hence the amount of money made if you sell 75 sandwiches is $111.25

To make $100 profit, the amount of sandwiches must you sell is given as:

`\begin{gathered} 100=5.15x-275 \\ 5.15x=100+275 \\ 5.15x=375 \\ x=(375)/(5.15) \\ x\approx72\text{sandwiches} \end{gathered}`

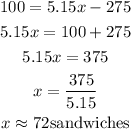
Hence 72 sandwiches must be sold to make a profit of $100