Part D
we have the function
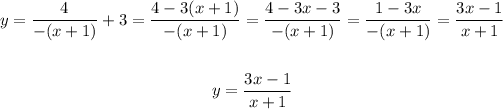
In this rational function
Remember that
The denominator cannot be equal to zero
so
The value of x cannot be equal to x=-1
At x=-1 there is a vertical asymptote
Find out a horizontal asymptote
Degree on Top is Equal to the Bottom
so
the horizontal asymptote is at y=3/1=3
Find out the intercepts
y-intercept (value of y when the value of x=0)
For x=0
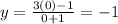
The y-intercept is (0,-1)
Find out the x-intercept (value of x when the value of y=0)
For y=0
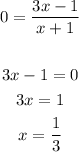
The x-intercept is (0.33,0)
With the given information
Graph the function
using a graphing tool
see the figure below