Question

8+7i/4-6iI need the answer and how to solve asap!

Answer 1

`(1)/(52)(-10\text{ + 76i) or }(1)/(26)(-5\text{ + 38i)}`

Step-by-step explanation

We are given the fraction of complex numbers:

`\frac{\text{8 + 7i}}{4\text{ - 6i}}`

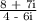
To simplify this, we will find the conjugate of the denominator and then multiply that with the numerator and denomiator.

The conjugate is gotten by changing the sign of the denominator. That is:

4 + 6i

So, we have:

`\begin{gathered} \frac{\text{8 + 7i}}{4\text{ - 6i}}\cdot\text{ }\frac{4\text{ + 6i}}{4\text{ + 6i}} \\ =\text{ }\frac{(8\text{ + 7i) (4 + 6i)}}{(4\text{ - 6i) (4 + 6i)}} \\ =\frac{(8\cdot\text{ 4) + (8 }\cdot\text{ 6i) + (7i }\cdot\text{ 4) + (7i }\cdot\text{ 6i)}}{(4\cdot\text{ 4) + (6i }\cdot\text{ 4) - (6i }\cdot\text{ 4) - (6i }\cdot\text{ 6i)}} \\ We\text{ know that i = }√(i),\text{ so i }\cdot\text{ i = -1:} \\ \Rightarrow\text{ }\frac{\text{ }32\text{ + 48i + 28i - 42}}{16\text{ + 24i - 24i + 36}} \\ =\text{ }\frac{-10\text{ + 76i}}{16\text{ + 36}}\text{ = }\frac{-10\text{ + 76i}}{52} \\ =\text{ }(1)/(52)(-10\text{ + 76i) or }(1)/(26)(-5\text{ + 38i)} \end{gathered}`

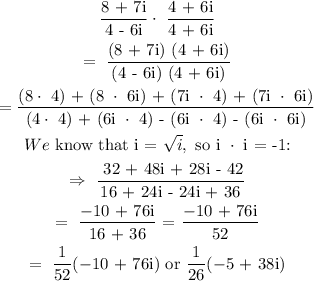
That is the answer.