Question

With cell phones being so common these days, the phone companies are all competing to earn business by offering various calling plans. One of them, Horizon, offers 700 minutes of calls per month for $45.99, and additional minutes are charged at 6 cents per minute. Another company, Stingular, offers 700 minutes for $29.99 per month, and additional minutes are 35 cents each. For how many total minutes of calls per month is Horizon’s plan a better deal?

Answer 1

For the Horizon offer

There is a cost of $45.99 for 700 minutes plus 6 cents for each additional minute

Since 1 dollar = 100 cents, then

6 cents = 6/100 = $0.06

If the total number of minutes is x, then

The total cost will be

`C_H=45.99+(x-700)0.06\rightarrow(1)`

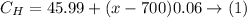
For the Stingular offer

There is a cost of $29.99 for 700 minutes plus 35 cents for each additional minute

35 cents = 35/100 = $0.35

For the same number of minutes x

The total cost will be

`C_S=29.99+(x-700)0.35\rightarrow(2)`

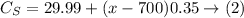
For Horizon to be better that means, it cost less than the cost of Stingular

Add 215.01 to both sides

`\begin{gathered} 3.99+215.01+0.06x<-215.01+215.01+0.35x \\ 219+0.06x<0.35x \end{gathered}`

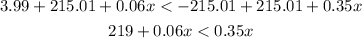
Subtract 0.06x from both sides

`\begin{gathered} 219+0.06x-0.06x<0.35x-0.06x \\ 219<0.29x \end{gathered}`

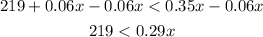
Divide both sides by 0.29 to find x

[tex]\begin{gathered} \frac{219}{0.29}<\frac{0.29x}{0.29} \\ 755.17Then x must be greater than 755.17

The first whole number greater than 755.17 is 756

The total minutes should be 756 minutes per month for Horizon's to be the better deal.