Question

A junk drawer at home contains eight pens four of which work what is the probability that a randomly grab three pens from the drawer and don’t end up with a pen that works express your answer as a fraction in lowest terms or decimal rounded to the nearest million

Answer 1

1/14

Step-by-step explanation:

The number of ways or combinations in which we can select x objects from a group of n can be calculated as:

`\text{nCx}=(n!)/(x!(n-x)!)`

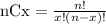
So, if we are going to select 3 pens from the drawer that contains 8 pens, the number of possibilities is:

`8C3=(8!)/(3!(8-3)!)=\frac{8!}{3!\cdot5!^{}}=56`

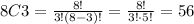
Then, if we didn't end up with a pen that works is because we select the three pens from the 4 that didn't work. In this case, the number of possibilities is:

`4C3=(4!)/(3!(4-3)!)=(4!)/(3!\cdot1!)=4`

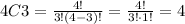
Therefore, the probability required is equal to the ratio of these quantities:

`P=(4)/(56)=(1)/(14)`

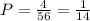
So, the answer is 1/14