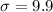By definition, the standard deviation is
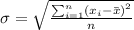
It seems hard so let's do it step by step, first, let's find the mean of the data
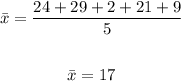
Now we have the mean value, let's do each value of the set minus the mean value

Now we have the difference between each element and the mean value, let's do the square of all values
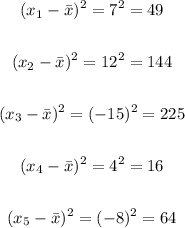
Now we have the square of the difference we sum them
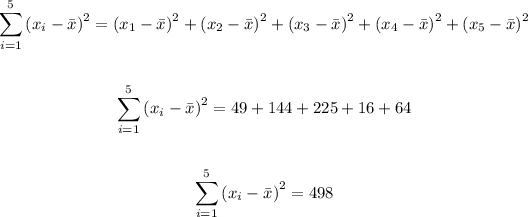
Now we have the sum we must divide by the number of elements, in that case, 5 elements
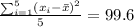
Now we take the square root of that value to have the standard deviation!
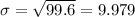
We write it using only one decimal the result would be
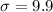
With no rounding.
Final answer: