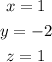Given
Solve the following system of linear equations.
x + 3y + z = - 4
2x – 4y – 3z = 7
3x – 3y + 4z = 13
Solution
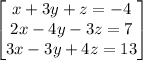
Substitute x= -4-3y-z
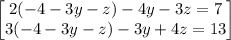
Simplify
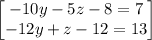
Make y the subject
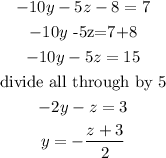
Now substitute
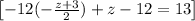
Simplify
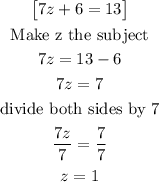
Now substitute z=1
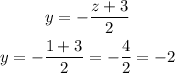
Finally, to find x
when z =1 and y =-2
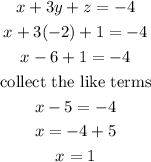
The final answer