Question

Name the ordered pair for a fourth point. Q. so that points P.Q.R. and S will be the vertices of a Given: Points P6.-1), R(0.-1) and S(4.-5) rectangle Response

Answer 1

to find Q we need to make 2 distance measure

that they fulfill these conditions

PS=RQ and RS=QP

the formula of distances between 2 points is

`\sqrt[]{(x2-x1)^2+(y2-y1)^2}`

Distance PS

`\begin{gathered} \sqrt[]{(6-4)^2+(-1-(-5))^2} \\ \\ PS=\sqrt[]{20} \end{gathered}`

Distance RQ

`\begin{gathered} \sqrt[]{(0-x)^2+(-1-y)^2} \\ \\ RQ=\sqrt[]{x^2+(1+y)^2} \end{gathered}`

where x and y are de coordinates of Q

Distance RS

`\begin{gathered} \sqrt[]{(0-4)^2+(-1-(-5))^2} \\ \\ RS=\sqrt[]{32} \end{gathered}`

Distance QP

`\begin{gathered} \sqrt[]{(x-6)^2+(y-(-1))^2} \\ \\ QP=\sqrt[]{(x-6)^2+(y+1)^2} \end{gathered}`

now solve the equals

PS=RQ

`\begin{gathered} \sqrt[]{20}=\sqrt[]{x^2+(1+y)^2} \\ 20=x^2+(1+y)^2 \end{gathered}`

RS=QP

`\begin{gathered} \sqrt[]{32}=\sqrt[]{(x-6)^2+(y+1)^2} \\ 32=(x-6)^2+(y+1)^2 \end{gathered}`

if I subtract the two equations I will get

`32-20=(x-6)^2-x^2`

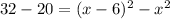
and i will solve to find x

`\begin{gathered} 12=-12x+36 \\ 12x=36-12 \\ x=(24)/(12) \\ \\ x=2 \end{gathered}`

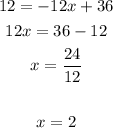
the value of x is 2, then I can replace x on any equation to find y

so replacing

`\begin{gathered} 20=x^2+(1+y)^2 \\ 20=(2)^2+y^2+2y+1 \\ y^2+2y+1-20+4=0 \\ y^2+2y-15=0 \end{gathered}`

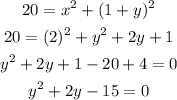
use factor to solve y

`\begin{gathered} y=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ \\ y=\frac{-2\pm\sqrt[]{4+60}}{2} \\ \\ y=(-2\pm8)/(2) \\ \\ y=-1\pm4 \end{gathered}`

then y will have two values

`\begin{gathered} y_1=-1+4=3 \\ y_2=-1-4=-5 \end{gathered}`

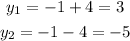
the real coordinate is y=3 because if is y=-5 the point dont form a rectangle

if x=2 and y=3 the point Q is (2,3)