Question

The area of a new wctabfle is 28m^2 and the length of the rectangle is 1m more than double the width. Find the dimensions

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Represent the sides of the rectangle

Let the length be represented by l

Let the width be represented by w

STEP 2: Interpret the statements in the question.

`\begin{gathered} length\text{ is 1m more than the double of the width:} \\ double\text{ of the width}\Rightarrow2w \\ 1m\text{ more than the double}\Rightarrow2w+1 \\ \therefore l=2w+1 \end{gathered}`

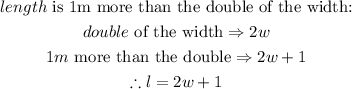
STEP 3: Equate the area of the rectangle to given measure

`\begin{gathered} Area=length* width \\ length=2w+1,width=w \\ Area=(2w+1)\cdot w=28 \\ By\text{ simplification,} \\ w(2w+1)=28 \end{gathered}`

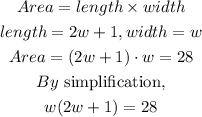
STEP 4: Solve for the width

`\begin{gathered} w(2w+1)=28 \\ By\text{ expansion,} \\ 2w^2+w=28 \\ Subtract\text{ 28 from both sides} \\ 2w^2+w-28=28-28 \\ 2w^2+w-8=0 \end{gathered}`

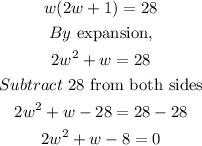
STEP 5: Solve the equation using quadratic formula

`quadratic\text{ formula}\Rightarrow(-b\pm√(b^2-4ac))/(2a)`

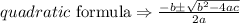
From the equation,

`a=2,b=1,c=-28`

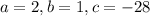
By substitution,

`\begin{gathered} w_(1,\:2)=(-1\pm√(1^2-4\cdot\:2\left(-28\right)))/(2\cdot\:2) \\ √(1^2-4*2(-28))=15 \\ By\text{ substitution,} \\ w_(1,\:2)=(-1\pm \:15)/(2\cdot \:2) \\ \mathrm{Separate\:the\:solutions} \\ w_1=(-1+15)/(2\cdot \:2),\:w_2=(-1-15)/(2\cdot \:2) \\ w=(-1+15)/(2*2)=(14)/(4)=(7)/(2)=3.5 \\ w=(-1-15)/(2\cdot\:2)=(-16)/(4)=-4 \end{gathered}`

Since the width cannot be negative, this means that the value of the width is 3.5m

STEP 6: Solve for the length

By substitution into the formula in step 2, we have:

`\begin{gathered} l=2w+1 \\ l=2(3.5)+1=8 \\ l=8 \end{gathered}`

Hence,

length = 8m

width = 3.5m