To find if any given point is a solution for the linear equation, simply plug in the x and y values given and check if the equality stands, as following:
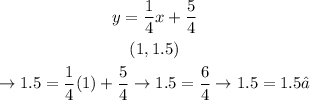
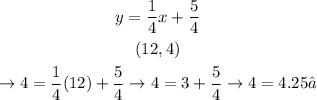
Thereby the answer is:
C. (1, 1.5) is a solution but (12, 4) is not
Now, to find the x-intercept just make y = 0 and clear x, as following:
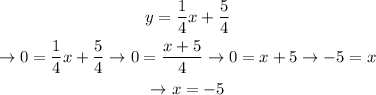
Therefore, the x-intercept is -5