Question

Which is an equation

Answer 1

An equation is 2 equivalent expressions/values that are represented by placing a “=“ (equal sign) between them

Answer 2

The slope is define as the rate of y coordinate with respect to the x coordinate.

`\text{Slope}=(y_2-y_1)/(x_2-x_1)`

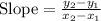
In the line D which is parallel to x axis has the constant y coordinate i.e

y = -3

So, for the numerator of the slop for line D is ( -3) - ( -3) = 0

Thus the slope will be express as :

`\begin{gathered} \text{Slope}=(y_2-y_1)/(x_2-x_1) \\ \text{Slope}=(-3-(-3))/(x_2-x_1) \\ \text{Slope}=(0)/(x_2-x_1) \\ \text{Slope = }0 \end{gathered}`

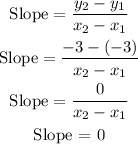
Thus the slope of line is 0

Now, for the line A :

The line A is parallel to y axis, that is only y coorsinates are changes x is at contant position.

i.e. x = -5

So, substitute the value in the expression for the slope :

Slope of line A is not define

In the line B and C, the coordinates of x and y aixs are changes countinously

thus thier slopes will be well define.

Answer : Slope of line A is not define.