Question

Translate the following statement into probability notation news X as the random variable

Answer 1

Let be x the random variable

a)

If we need the probability of x when it is not more than a, we can write it using inequalities as below

`x\le a`

Notice that x can be equal to a but no more than a

And the probability is then

`P(x\le a)`

So, the answer to question a) is the 4th option

b) 'x being at least a' can be written using inequalities in this way:

`x\ge a`

Notice that x can be equal to a, but not less than a

Therefore, the probability is given by the expression

`P(x\ge a)`

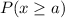
So, the answer to question b) is the last option