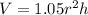Answer:
V = 1.05r²h
Step-by-step explanation:
The expression ''the volume V of a cone varies jointly as the square of the radius of the base,r, and the height, h'' can be represented as:

Where the k is a constant.
So, replacing V = 285, r = 4, and h = 17, we get:

Solving for k, we get:

So, the equation of the joint variation is: