Given:
The diameter of the hemisphere is 15 inches.
To find:
The surface area of a hemisphere.
Step-by-step explanation:
The radius of the hemisphere is
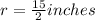
Using the formula of the surface area of a hemisphere,
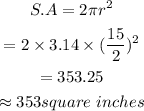
Final answer:
The surface area of the hemisphere is 353 square inches.