Question

The question is in the picture. Is B one of the right answers? Is B the only right answer?

Answer 1

To answer this question we first need to notice that the sequence of dots increases by a factor of 3 in each drawing. This means that this is a geometric sequence with common ratio 3.

We know that the nth term of a geometric sequence is given by:

`a_n=a_1r^(n-1)`

where a1 is the first term and r is the common ratio. In this case a1=3 and r=3, hence the nth term is:

`a_n=3(3)^(n-1)`

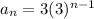
Now, if we add the first 15 stages, this means that we are adding the first 15 terms of the sequence, then our sum will be of the form:

`\begin{gathered} a_1+a_2+a_3+a_4+\cdot\cdot\cdot+a_(15)_{} \\ =3(3)^(1-1)+3(3)^(2-1)+3(3)^(3-1)+3(3)^(4-1)+\cdot\cdot\cdot+3(3)^(15-1) \\ =3(3)^0+3(3)^1+3(3)^2+3(3)^3+\cdot\cdot\cdot+3(3)^(14) \\ =3(3^0+3^1+3^2+3^3+\cdot\cdot\cdot+3^(14)) \\ =3(1^{}+3^1+3^2+3^3+\cdot\cdot\cdot+3^(14)) \end{gathered}`

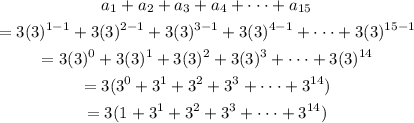
Then we notice that option a is a correct expression for the sum of the first 15 stages.

We also know that the sum of the first nth terms of a geometric sequence is given by:

`(a_1(1-r^n))/(1-r)`

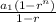
plugging the values we know, we have that:

`3((1-3^(15)))/(1-3)`

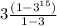
Therefore option d is also a correct expression for the sum of the first 15 stages.