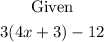
By following the order of operations, we need to evaluate first the multiplication of 3 and (4x+3) by using the distributive property.
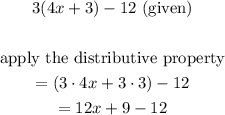
After that, combine like terms, we see from the previous solution that 12x has no other like terms, and it will remain as is. 9 and 12 however are both constant, and should be simplified
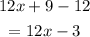
Therefore, 3(4x+3) - 12, when simplified is 12x - 3.